Paper Accepted for Publication in Finite Elements in Analysis and Design
Amrita Francis, Alejandro Ortiz-Bernardin, Stéphane P. A. Bordas, Sundararajan Natarajan, “A MINI element over star convex polytopes”
ABSTRACT
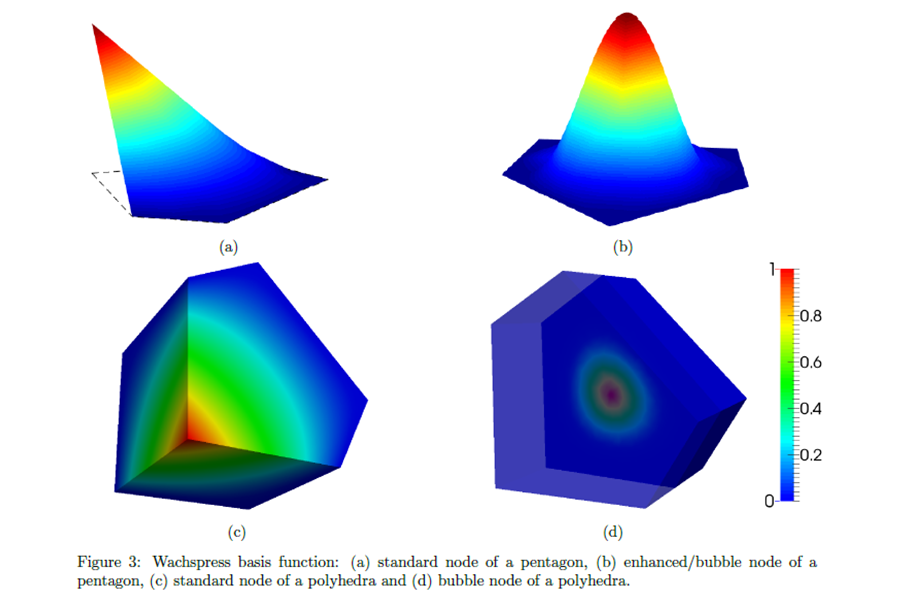
In this paper, we extend the concept of MINI element over triangles to star convex arbitrary polytopes. This is achieved by employing the volume averaged nodal projection (VANP) method over polytopes in combination with the strain smoothing technique. Within this framework, the dilatation strain is projected onto the linear approximation space, thus resulting in a purely displacement based formulation. The stability is ensured by enhancing the displacement field with bubble basis functions. The salient features of the proposed method are two fold: the VANP alleviates the locking phenomenon and the strain smoothing suppresses the need to compute the derivative of the basis functions, thus reducing the computational burden. Various benchmark problems in two and three dimensions are numerically solved to demonstrate the robustness, accuracy and the convergence properties of the proposed framework.