A volume-averaged nodal projection method for the Reissner-Mindlin plate model
- Post by: alejandro
- July 31, 2018
- Comments off
Computer Methods in Applied Mechanics and Engineering
Vol. 341, pp. 827-850, 2018
A. Ortiz-Bernardin, P. Köbrich, J. S. Hale, E. Olate-Sanzana, S. P. A. Bordas, S. Natarajan
Abstract
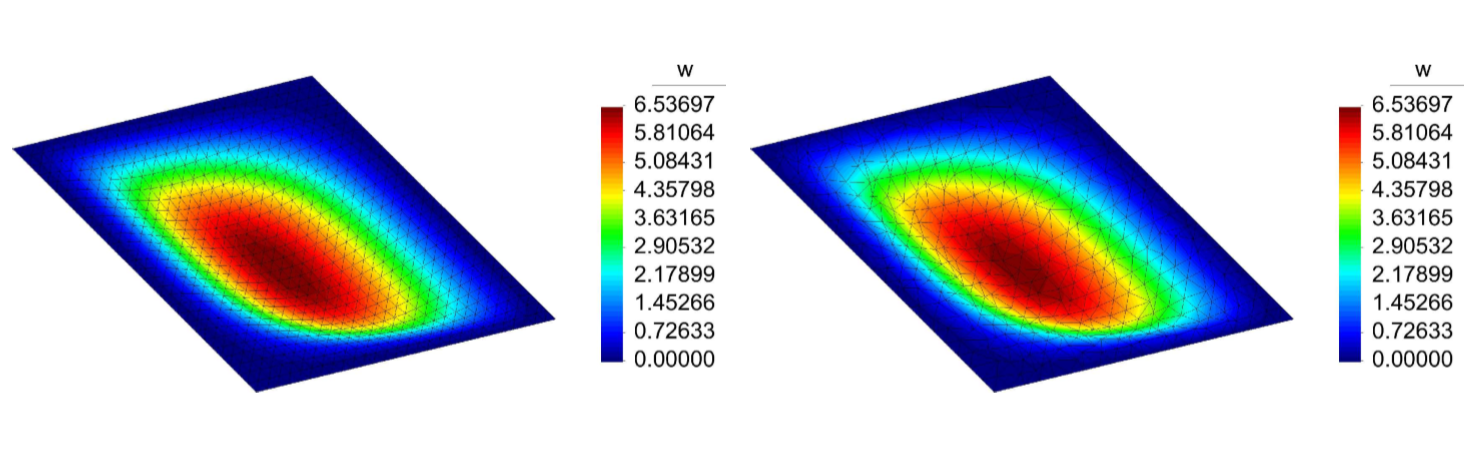
We introduce a novel meshfree Galerkin method for the solution of Reissner-Mindlin plate problems that is written in terms of the primitive variables only (i.e., rotations and transverse displacement) and is devoid of shear-locking. The proposed approach uses linear maximum-entropy approximations and is built variationally on a two-field potential energy functional wherein the shear strain, written in terms of the primitive variables, is computed via a volume-averaged nodal projection operator that is constructed from the Kirchhoff constraint of the three-field mixed weak form. The stability of the method is rendered by adding bubble-like enrichment to the rotation degrees of freedom. Some benchmark problems are presented to demonstrate the accuracy and performance of the proposed method for a wide range of plate thicknesses.
Keywords: meshfree methods; maximum-entropy approximation; Reissner-Mindlin plate; shear-locking; VANP method.
Original Journal Article: https://doi.org/10.1016/j.cma.2018.07.023
Personal preprint:
NOTICE: this is the author’s version of a work that was accepted for publication. Changes resulting from the publishing process, such as peer review, editing, corrections, structural formatting, and other quality control mechanisms may not be reflected in this document.
